Circumference Of A Circle Formula Using Radius

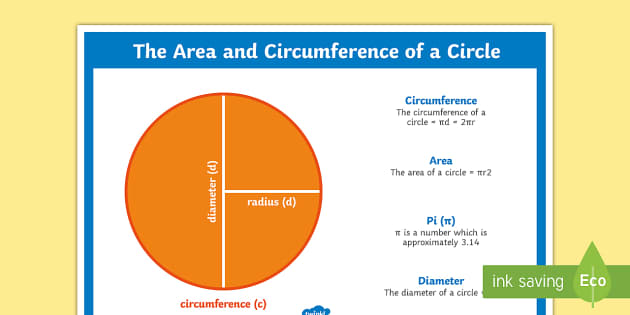
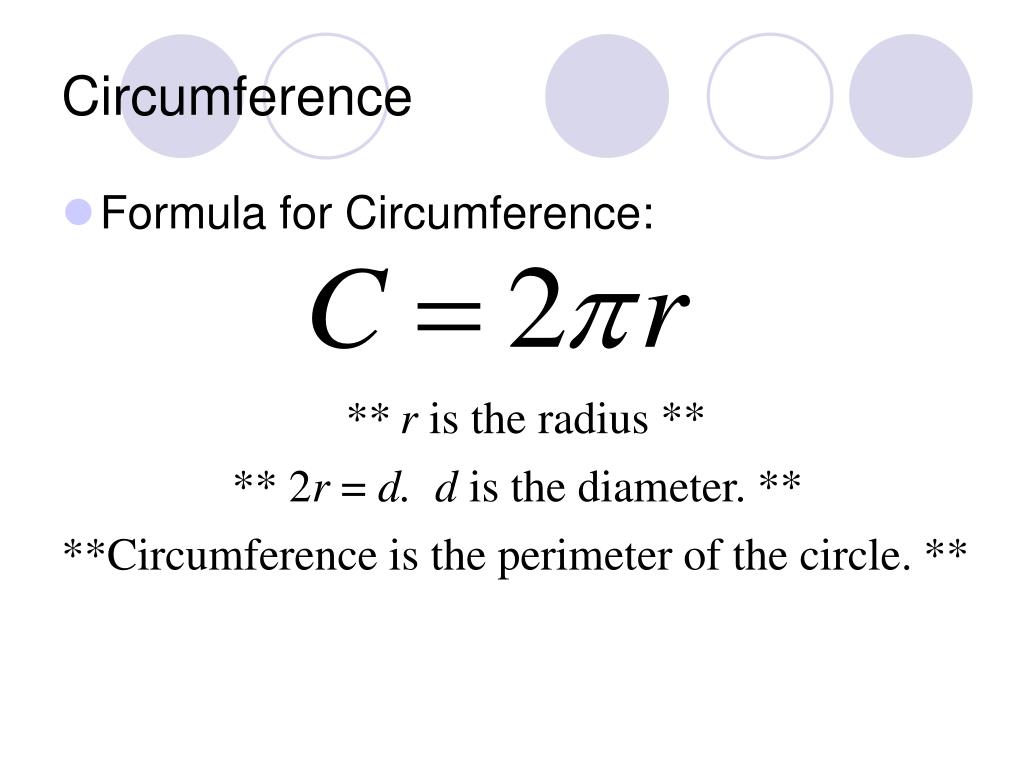
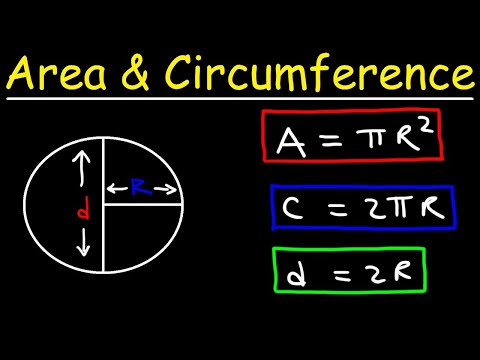
The circumference of a circle of radius $r$ is $2\pi r$. This well known formula is taken up here from the point of view of similarity. It is important to note in this task that the definition of $\pi$ already involves the circumference of a circle, a particular circle.

In order to show that the ratio of circumference to diameter does not depend on the size of the circle, a similarity argument is required. Two different approaches are provided, one using the fact that all circles are similar and a second using similar triangles. This former approach is simpler but the latter has the advantage of leading into an argument for calculating the area of a circle. Calculating areas and circumferences of circles plays an important role in almost all field of science and real life. For instance, formula for circumference and area of a circle can be applied into geometry.

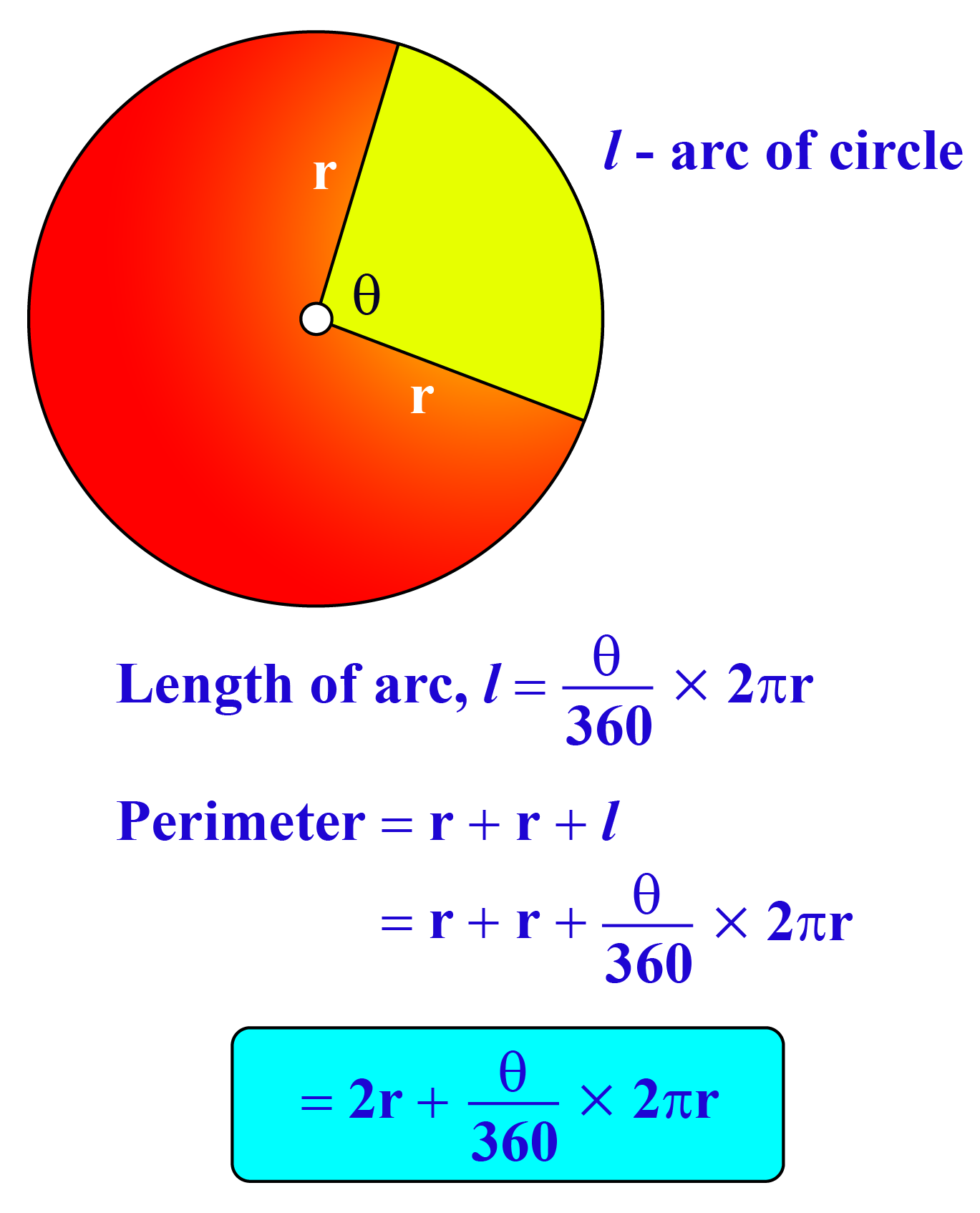
They are used to explore many other formulas and mathematical equations. An arch length is a portion of the circumference of a circle. The ratio of the length of an arc to the circumference is equal to the ratio of the measure of the arc to $360$ degrees. A sector of a circles is the region bounded by two radii of the circle and their intercepted arc.

Area Of A Circle Formula Using Radius For any other value for the length of the radius of a circle, just supply a positive real number and click on the GENERATE WORK button. They can use these methods in order to determine the area and lengths of parts of a circle. When the formula for calculating the circumference of a circle is used, the circle's radius is considered. As a result, the radius or diameter value must be known to calculate the circle's perimeter or circumference.

We'll teach you the key circumference formulas you need to figure out the circumference of a circle when you know either the diameter or radius. The learning outcome of this article is that one can calculate the circumference of circular objects and circular paths. The circumference of a circle is the measurement around a circle's edge. It can be compared to finding the perimeter of a shape . If you were to cut a circle and lay the outline flat, the length of the line it created would be its circumference.

The circumference can be measured in any unit or system that traditionally measures length - imperial (inches, feet, etc.) or metric (centimeters, meters, etc.). Whichever unit the radius is measured in will also be the unit the circumference is calculated in. The formula for the circumference of a circle in terms of its radius comes from the definition of the constant . The constant is defined as the ratio of any circles circumference divided by its diameter. This is illustrated in the figure below.

This first argument is an example of MP7, Look For and Make Use of Structure. The key to this argument is identifying that all circles are similar and then applying the meaning of similarity to the circumference. The second argument exemplifies MP8, Look For and Express Regularity in Repeated Reasoning. Here the key is to compare the circle to a more familiar shape, the triangle.

The first solution requires a general understanding of similarity of shapes while the second requires knowledge of similarity specific to triangles. Two formulas are used to find circumference, C, depending on the given information. Both circumference formulas use the irrational number Pi, which is symbolized with the Greek letter, π. Pi is a mathematical constant and it is also the ratio of the circumference of a circle to the diameter. This concept can be of significance in geometry, to find the perimeter, area and volume of solids.

The circumference of any form specifies the route or border that surrounds it in mathematics. In other terms, the circumference is also known as the perimeter, and it is used to determine the length of a shape's contour. Is called the circumference and is the linear distance around the edge of a circle. The circumference of a circle is proportional to its diameter, d, and its radius, r, and relates to the famous mathematical constant, pi (π). Does calculating circumference have you running in circles? Our circumference calculator is an easy way for you to find the circumference of any circular object.

To make sure you are measuring the diameter correctly, it should be the biggest measurement you can get. If by moving the measurement instrument slightly you get a bigger diameter size, then go with that. Although the circumference of a circle is its length, it cannot be calculated with the help of a ruler like it is usually done for other polygons. This is because a circle is a curved figure. Therefore, to calculate the circumference of a circle, we apply a formula that uses the radius or the diameter of the circle and the value of Pi (π). Once again in this example, we're given the radius of the circle.

Although it's not a clean number like our previous example, but we can still simply plug the number directly into the formula like what we did above. Be aware of the units that this circle's radius is given in and remember to give your final answer in the same unit. In this question, we find that the circumference is equalled to 53.41m. The distance from the centre to the outer line of the circle is called a radius. It is the most important quantity of the circle based on which formulas for the area and circumference of the circle are derived. Twice the radius of a circle is called the diameter of the circle.

The diameter cuts the circle into two equal parts, which is called a semi-circle. When we use the formula to calculate the circumference of the circle, then the radius of the circle is taken into account. Hence, we need to know the value of the radius or the diameter to evaluate the perimeter of the circle. The diameter of the circle is the largest chord and is passing through the center of the circle. The circumference of the circle is the length of the outer boundary of the circle.

Both the diameter and the circumference are lengths and have linear units for measurement. Also, the circumference of the circle is equal to the product of the diameter and the constant pi. The perimeter of a circle is the same as the circumference of a circle.

It is the total length of the outer boundary of the circle. The perimeter or circumference of a circle is the product of the constant pi and the diameter of the circle. It is a linear one-dimensional quantity and has units such as m, inch, cms, feet.

It looks like you calculated the area of a circle using a radius of 2; in this figure, the radius of each circle is 1. To find the area of the figure, imagine the two semi-circles are put together to create one circle. Then calculate the area of the circle and add it to the area of the square.

Area and circumference of circle calculator uses radius length of a circle, and calculates the perimeter and area of the circle. It is an online Geometry tool requires radius length of a circle. Using this calculator, we will understand methods of how to find the perimeter and area of a circle.

One can also find the area of a circle if the circumference is given. For this first, we have to divide the circumference by π and then by 2. Then put the radius in the area formula to calculate the area. The radius of the circle is taken into account when using the formula to determine the circle's circumference. As a result, to calculate the perimeter of a circle, we must first determine the radius or diameter.

Write the formula for circumference of circle with radius r. The Greek letter p (pronounced as "pie") is used to describe this number. It stands for the ratio between the circumference of any circle and its diameter, and it's true for all circles.

This means that any circle's circumference will be about 3.14 times the length of its diameter. There are two ways of finding the perimeter or circumference of a circle. The first formula involves using the radius, and the second involves using the diameter of a circle.

It is important to note that both two methods yield the same result. The distance around a polygon, such as a square or a rectangle, is called the perimeter . On the other hand, the distance around a circle is referred to as the circumference . Therefore, the circumference of a circle is the linear distance of an edge of the circle.

For example, the length of a 180 degree arc must be half the circumference of the circle, the product of pi and the radius. The length of any arc is equal to whatever fraction of a full rotation the arc spans multiplied by the circumference of the circle. A 45 degree arc, for example, spans one-eighth of a full rotation, and is therefore equal to one-eighth the circumference of that circle. The length of an arc of n degrees equals (n/360) times the circumference. This lesson has provided you with lots of information the circumference of circles and a way to find any the measure of any one part if you have another measurement.

Along the way, you also learned a little geography and history, which may also come in handy to you. Pi (π) is a special mathematical constant; it is the ratio of circumference to diameter of any circle. Now that you know how to calculate the circumference and area of a circle, you can use this knowledge to find the perimeter and area of composite figures.

The diameter of any circle is two times the length of that circle's radius. The calculation of the circumference of a circle is simple, and you just have to multiply its diameter with the constant pi (3.142). To get the circumference from radius, multiply 2 x radius by pi. The radius of a circle is the distance between its centre and its outside line. It is the most significant quantity in the circle, from which the formulae for the circle's area and circumference are generated.

A circle's diameter is equal to twice its radius. A semicircle is created when the diameter divides a circle into two halves. A circle is divided into congruent sectors . The sectors are pulled out of the circle and are arranged as shown in the middle diagram.

The length across the top is half of the circumference. When placed in these positions, the sectors form a parallelogram. The larger the number of sectors that are cut, the less curvy the arcs will appear and the more the shape will resemble a parallelogram. As seen in the last diagram, the parallelogram ca be changed into a rectangle by slicing half of the last sector and placing it to the far left. If you know the circumference, radius, or diameter of a circle, you can also find its area. Area represents the space enclosed within a circle.

It's given in units of distance squared, such as cm2 or m2. Acircleis a closed round shape with all the points equidistant from a fixed point known as the centre. The circle's circumference is measured in length units such asmetresandcentimetres. As stated before, the perimeter or circumference of a circle is the distance around a circle or any circular shape. The circumference of a circle is the same as the length of a straight line folded or bent to make the circle. The circumference of a circle is measured in meters, kilometers, yards, inches, etc.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations. Worksheetto calculate circumference of circle when given diameter or radius.

Suppose you know that the circumference of a circle is 20 centimeters and you want to calculate the radius. Just plug the value for the circumference into the equation and solve. Remember that pi is approximately equal to 3.14. Lauren is planning her trip to London, and she wants to take a ride on the famous ferris wheel called the London Eye.

While researching facts about the giant ferris wheel, she learns that the radius of the circle measures approximately 68 meters. What is the approximate circumference of the ferris wheel? And as Math Open Reference states, the formula takes the circumference of the entire circle (2πr).

It reduces it by the ratio of the degree measure of the arc angle to the degree measure of the entire circle . The term circumference is used when measuring physical objects, as well as when considering abstract geometric forms. Π shows the ratio of the perimeter of a circle to the diameter. Therefore, when you divide the circumference by the diameter for any circle, you obtain a value close enough to π.